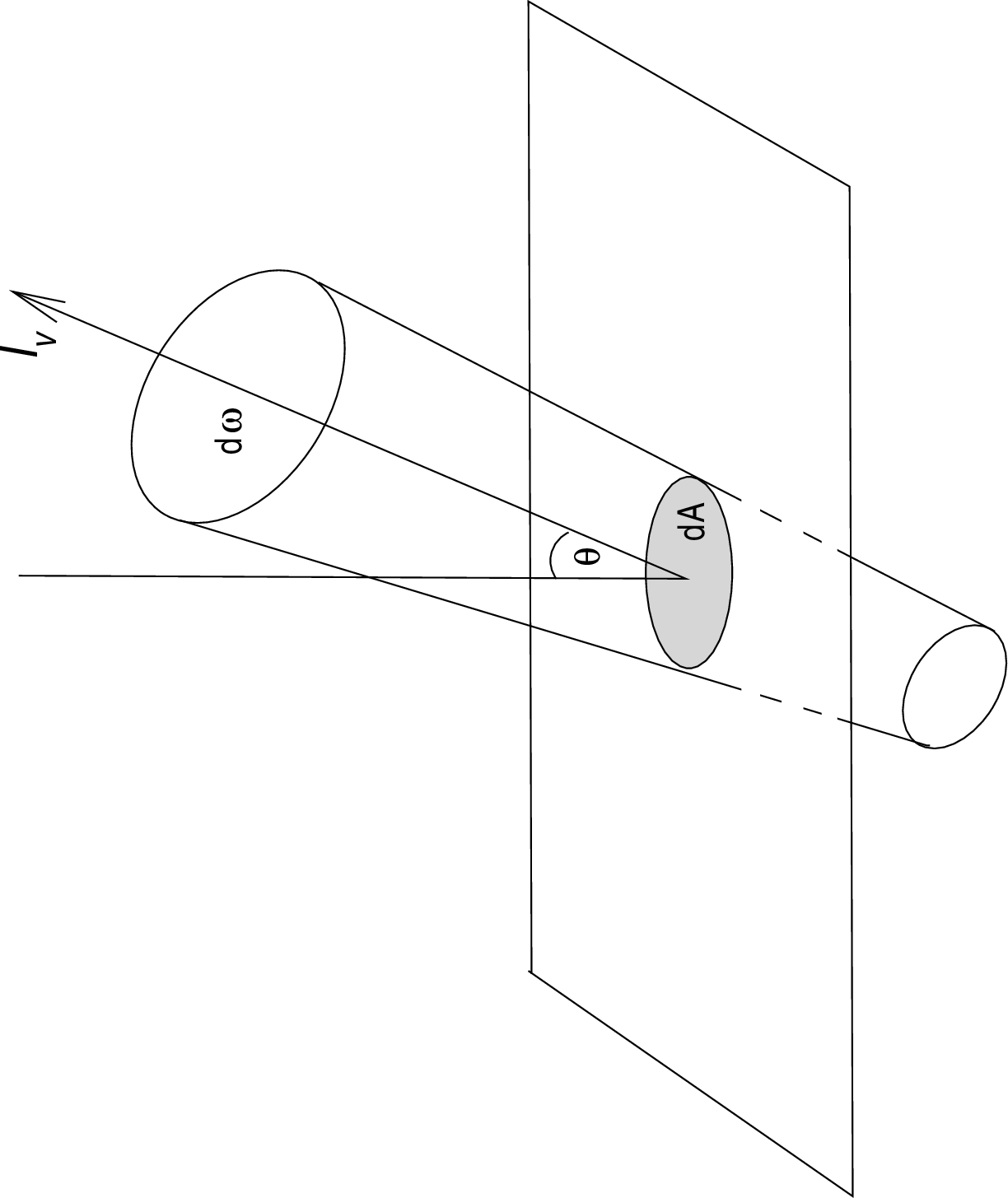
This section recapitulates some of the basic concepts and equations of radiation theory. Further details can be found in any standard introductory textbook on astrophysics. One such classic text is Unsöld’s The New Cosmos[74]. However, there are numerous suitable textbooks. Assume some radiation passing through a surface and consider an element of the surface of area (Figure 1). Some of the radiation will leave the surface element within a beam of solid angle at an angle to the surface. The amount of energy entering the solid angle within a frequency range in a time will be:
| (1) |
where is the specific intensity of radiation at the frequency in the direction of the solid angle, with dimensions of .

The intensity including all possible frequencies, the total intensity , can be obtained by integrating over all frequencies:
| (2) |
From an observational point of view we are generally more interested in the energy flux or flux () and the flux density ()1. Flux density gives the power of the radiation per unit area and hence has dimensions of or . Observed flux densities are usually extremely small and therefore (especially in radio astronomy) flux densities are often expressed in units of the Jansky (Jy), where 1 Jy .
If we consider a star as the source of radiation, then the flux emitted by the star into a solid angle is , where is the flux density observed at a distance from the star (it is also usual to refer to the total flux from a star as the luminosity, ). If the star radiates isotropically then radiation at a distance will be distributed evenly on a spherical surface of area and hence we get the relationship:
| (3) |
The situation is slightly more complicated for an extended luminous object such as a nebula or galaxy. The surface brightness is defined as the flux density per unit solid angle. The geometry of the situation results in the interesting fact that the observed surface brightness is independent of the distance of the observer from the extended source. This slightly counter-intuitive phenomenon can be understood by realising that although the flux density arriving from a unit area is inversely proportional to the distance to the observer, the area on the surface of the source enclosed by a unit solid angle at the observer is directly proportional to the square of the distance. Thus the two effects cancel each other out.
1You should be aware - and beware - that different authors define the terms flux density, flux and intensity differently, and they are sometimes used interchangeably!